| |||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||
CHAPTER V
RESULTS AND DISCUSSION
GREYBULL RIVER STUDY AREA
The period of analysis to be discussed in this thesis for the Greybull River study reach extends from July 17, 1992 to September 30, 1992.
The Greybull River study reach was separated into two sub-study reaches for input into the J349 model. Diversion flow data from Meeteetsee to the Arnold Ditch made up the first reach (Reach 1) while flow data from the Arnold Ditch to the Farmers and Bench Canals was the second study reach (Reach 2).
Conveyance loss analysis was also separated into monthly trial periods. Monthly periods were chosen since the model, as discussed earlier, allowed for only twenty-five changes in diversion values. Monthly study periods also allowed for a comparison between conveyance loss values on a monthly basis. The first study period analyzed was July of 1992. The model was calibrated, as discussed in Chapter 4, to the point where the predicted downstream hydrograph calculated by the J349 model simulated the actual downstream hydrograph for the month of July (Figure 7). Table 3 presents the final stream and aquifer parameters determined from the calibration process. Final transmissivity for the aquifer determined by calibration was estimated to be 14,000 ft2/day. Transmissivity was estimated for the Greybull River area to range between 2,000 ft2/day and 18,000 ft2/day by Libra, Doremus and Goodwin (1981).
Figure 7. Greybull River (July 1992) Calibration Comparison of Actual
Flows and Modeled Flows.

In the calibration process, it was found that transmissivity and storativity had a great influence on conveyance losses calculated by the J349 model. Figure 8 illustrates the effect of varying transmissivity and storativity on the conveyance losses predicted by the model using July flow data. Transmissivity was increased from 50 to 20,000 ft2/day for three different storage coefficients. Increase in transmissivity between 500 and 2000 ft2/day showed the most marked increase in conveyance loss.
TABLE 3
FINAL MODELING PARAMETERS FOR THE GREYBULL RIVER STUDY REACH.
INPUT PARAMETER VALUE ________________________________________________________________________ REACH 1 REACH 2 Transmissivity (ft2/day) 14,000 14,000 Storativity 0.45 0.45 Aquifer Width (ft) 2155 3265 Wave Dispersion (ft2/sec) 475.0 475.0 Wave Celerity (ft/sec) 7.3 7.3 Base Flow (cfs) 50.0 50.0 Length of Channel (miles) 13.6 14.4 Length'of Alluvium (miles) 11.5 12.3 Time of Travel (hrs) 4 4 _______________________________________________________________________
Figure 8. Greybull River Modelling Results, Conveyance Loss versus
Transmissivity
for Three Different Storage Coefficients.
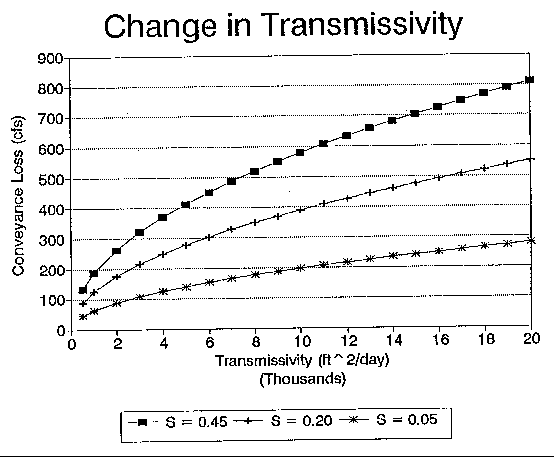
Increases in transmissivity between 2000 and 20,000 ft2/day shows successively smaller increases in conveyance loss as transmissivity was increased. The plot (Figure 8) illustrates, for example, that given an increase in transmissivity from 2000 to 4000 ft2/day and a storage coefficient of 0.20, the conveyance loss increases from 176 cfs to 248 cfs, an increase of forty-one percent.
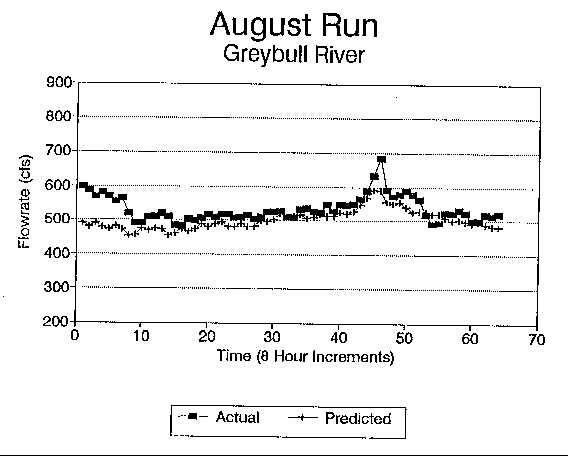
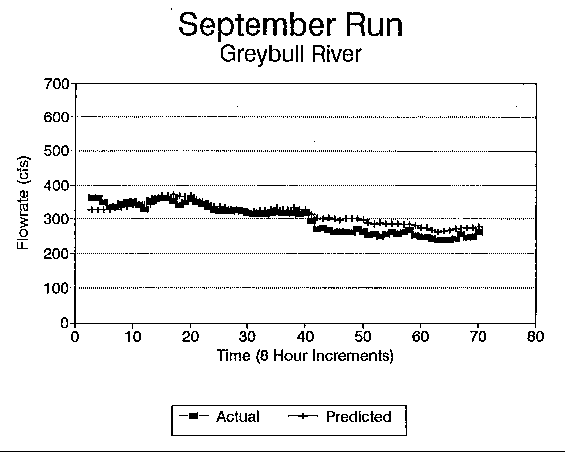
Streamflow data for the months of August and September were then modelled using the same stream and aquifer parameters that accurately simulated flow for the month of July. Predicted flow calculated by the computer program versus the actual flow measured for August and September are illustrated on Figures 9 and 10, respectively.
Figures 7, 9 and 10 illustrate that for several time increments near the start of the month, the model is inaccurate in predicting actual flow. Farber (1992) thoroughly discusses and explains the reasons for this inaccuracy. The main cause is due to the models' inability to obtain closure between streamflow, diversions and bank storage during the initial time steps (model needs a start up period to cause the model to synthesize correctly).
These figures also reveal that for July when flow values exceed 1000 cfs, the model consistently predicts a flowrate significantly (up to eighteen percent) lower than actually existed. Throughout the study period, approximately 2000 acre-feet (af) of water was not accounted for by the models predicted flow. This is approximately 9.7% of the total flow for the July simulation period.
In August, when flowrates ranged between 500 and 600 cfs, the model predicts flows lower than actually existed. Predicted values were up to eighteen percent lower than actual flow data for the first several time increments at the beginning of the simulation, and then stayed within five percent for the remainder of the study period. Approximately 1380 af of water is unaccounted for by the models predicted flow, or about 9.1% of total flow for the August simulation period.
The plot of September data shows that as actual flow data dips below 300 cfs, the model predicts flows higher than actually existed. Modelled flow data ranged between zero and ten percent higher than actual data. For the September study period, approximately 850 af of water, or 6.3% of total flow was not accounted for by the model.
In general, Figures 7, 9 and 10 show that the model can produce output that accurately simulates actual flow data for the Greybull River. Together with research conducted on the J349 program by Farber (1992), and the ability of the model to simulate actual flow data for the Greybull River, conveyance losses as calculated by the model are felt to be reasonably accurate for this study reach.
Figure 9. Greybull River (August 1992) Calibration Comparison of Actual Flows and Modeled Flows.
With the model theoretically calculating conveyance loss within accuracy limits of most gaged measurements, it is possible to determine an incremental conveyance loss for increases in flowrate. This is accomplished with the model by coding an upstream hydrograph with a constant flowrate into the working model for a one day period and letting the model determine a total conveyance loss for that upstream hydrograph as it passes down the river reach. The upstream hydrograph is then increased by increments of 50 cfs and the total conveyance loss for the new upstream hydrograph is determined. This process is repeated until the upstream hydrograph is at the highest expected flowrate for which conveyance loss estimates are generally needed. This procedure was performed for all three study months for the Greybull River study reach.
Figure 10. Greybull River (September 1992) Calibration Comparison of Actual Flows and Modeled Flows.
The daily diversion rate used in this process is the average daily diversion rate. An average daily diversion rate for each month is used in an effort to simulate conveyance loss for that month assuming that the diversions are representative of the same time periods for other years.
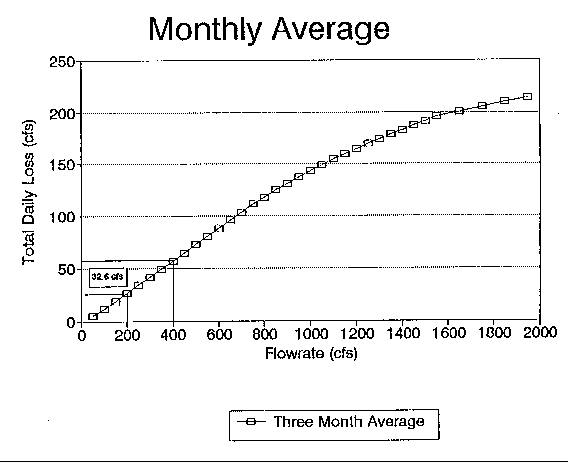
The results for the conveyance loss determination for each month and the average of all three months are shown in Table 4. The numbers indicate the total conveyance loss incurred in the Greybull River for a given flowrate for one day. Figure 11 illustrates a comparison between monthly conveyance losses. This figure (Figure 11) shows that total conveyance loss rises sharply initially and then levels off as the flowrate approaches 1500 cfs. Also there is little change in total conveyance loss between any month. Table 5 indicates the percent difference for each months total conveyance loss with respect to the three month average for each flowrate considered. With the exception of flowrates ranging from 50 to 150 cfs, all values are within ten percent of the three month average. Figure 12 illustrates the three month average of total conveyance losses, and is a reasonable representation of what should probably be used as the basis for determining incremental conveyance loss.
Conveyance losses determined using this method range from 0.40% to 0.62% per river mile of the incremental flow increase. This compares favorably with losses determined by Hanlin (1988), which were in the range of 0.3% to 4.7% loss per river mile. Losses determined for the Greybull River by the J349 model also fell within the 0.34% to 1.66% loss per river mile determined by Pahl (1985) for several streams in Wyoming.
The process for determining incremental conveyance loss involves using Figure 12 to determine the difference in total conveyance loss for two given flowrates. This difference in total conveyance loss is called an incremental conveyance loss. An example of the method used to determine this conveyance loss using Figure 12 is demonstrated on Figure 13. Figure 13 illustrates that at a flowrate of 400 cfs there is a total conveyance loss of 57.8 cfs, and at a flowrate of 200 cfs there is a total conveyance loss of 25.2 cfs. The incremental conveyance loss for an increase from 200 to 400 cfs for one day is 32.6 cfs.
TABLE 4
MONTHLY TOTAL CONVEYANCE LOSS FOR GREYBULL RIVER STUDY AREA
__________________________________________________________________________________________
July August September Average
Flowrate Loss Loss Loss Loss
(cfs) (cfs) (cfs) (cfs) (cfs)
50 5.65 3.94 4.76 4.78
100 13.26 8.74 12.04 11.36
150 20.91 16.01 19.64 18.85
200 28.51 23.49 27.3 26.43
250 36.3 31.07 34.88 34.08
300 44.13 38.72 42.53 41.79
350 51.96 46.73 50.2 49.63
400 59.79 54.02 58.03 57.26
450 67.62 61.67 65.87 65.05
500 75.46 69.32 73.7 72.83
550 83.29 76.97 81.53 80.60
600 91.14 84.62 89.37 88.38
650 98.83 92.47 97.14 96.15
700 106.25 100.15 104.72 103.71
750 113.33 107.61 111.92 110.95
800 120.11 114.73 118.79 117.88
850 126.56 121.58 125.4 124.51
900 132.78 128.11 131.7 130.86
950 138.72 134.33 137.75 136.93
1000 144.42 140.36 143.55 142.78
1050 149.93 146.08 149.15 148.39
1100 155.26 151.63 154.55 153.81
1150 160.44 157.01 159.79 159.08
1200 165.44 162.19 164.86 164.16
1250 170.3 167.26 169.78 169.11
1300 175.03 172.12 174.56 173.90
1350 179.6 176.9 179.19 178.56
1400 184.05 181.46 183.7 183.07
1450 188.41 185.96 188.08 187.48
1500 192.65 190.31 192.37 191.78
1550 196.05 194.46 195.82 195.44
1650 201.08 199.73 200.92 200.58
1750 205.7 204.58 205.63 205.30
1850 210.11 209.06 210.05 209.74
1950 214.31 213.39 214.3 214.00
__________________________________________________________________________________________
The incremental conveyance loss determined by the preceding procedure can be used to determine an incremental conveyance loss for each following day, assuming that the flowrate remains constant. Table 6 presents the results of running the J349 model to determine total conveyance loss, for between one and ten days with a constant flowrate of 500 cfs. A flowrate of 500 cfs was used because it falls within the 300 to 600 cfs range at which the river was flowing for most of the summer. This analysis was also performed at flowrates of 1000 and 1500 cfs for comparison. The results for flowrates of 1000 and 1500 cfs varied less than five percent on a day to day comparison with the 500 cfs flow.
Figure 11. Greybull River Modeling Results Comparison Between the Monthly Conveyance Losses.
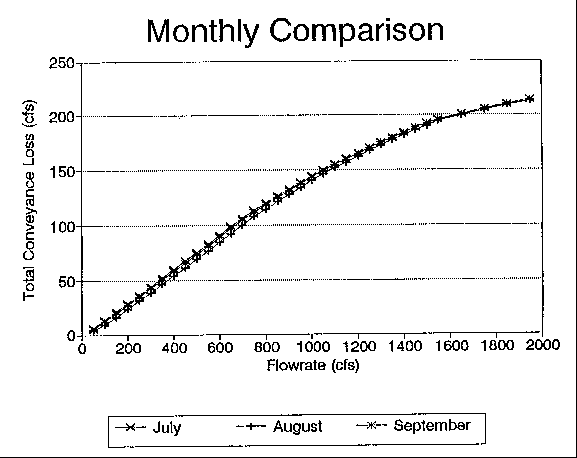
Table 6 gives the daily conveyance loss for that given flowrate for the first day and the following ten days. The table also indicates the percent of the original conveyance loss determined for each of the days. The data in this table is illustrated on Figure 14. Figure 14 indicates that conveyance loss on the second day is nearly identical to the loss on the first day. By the third day, however, the conveyance loss drops sharply to approximately 70% of the original conveyance loss. From the third day on, the percentage drops gradually and levels off at approximately 35% of the original conveyance loss by the 10th day.
TABLE 5
PERCENT DIFFERENCE OF MONTHLY CONVEYANCE LOSS FROM THREE MONTH
AVERAGE
______________________________________________________________________________________________________
Flowrate July August September
(cfs) (%) (%) (%)
50 18.12 17.63 0.49
100 16.76 22.78 6.02
150 10.91 15.08 4.17
200 7.86 11.13 3.28
250 6.50 8.84 2.34
300 5.59 7.35 1.76
350 4.69 5.84 1.15
400 4.38 5.69 1.31
450 3.95 5.20 1.26
500 3.62 4.82 1.20
550 3.34 4.50 1.16
600 3.13 4.25 1.12
650 2.79 3.82 1.03
700 2.45 3.43 0.98
750 2.14 3.01 0.87
800 1.89 2.67 0.77
850 1.64 2.36 0.71
900 1.46 2.10 0.64
950 1.30 1.90 0.60
1000 1.15 1.69 0.54
1050 1.04 1.55 0.51
1100 0.94 1.42 0.48
1150 0.85 1.30 0.45
1200 0.78 1.20 0.42
1250 0.70 1.10 0.39
1300 0.65 1.03 0.38
1350 0.58 0.93 0.35
1400 0.54 0.88 0.34
1450 0.49 0.81 0.32
1500 0.46 0.76 0.31
1550 0.31 0.50 0.19
1650 0.25 0.42 0.17
1750 0.19 0.35 0.16
1850 0.18 0.32 0.15
1950 0.14 0.29 0.14
___________________________________________________________________________________________________
Figure 12. Greybull River Modeling Results Showing Monthly Average of Total Conveyance Loss versus Flowrate.
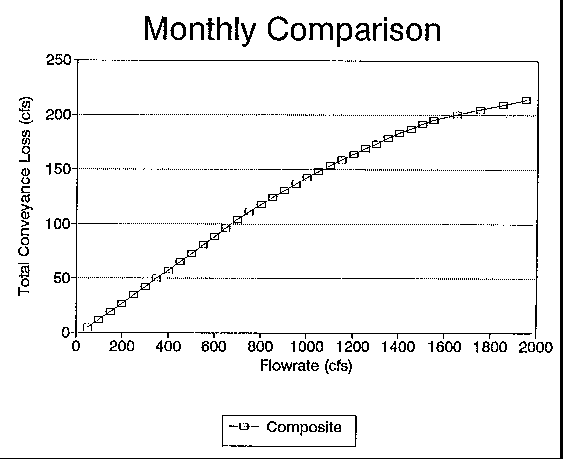
TABLE 6
TOTAL CONVEYANCE LOSS DETERMINED BY J349 MODEL AT A CONSTANT
FLOWRATE FOR ONE TO TEN DAYS
Day Total Conveyance Percent of Loss (cfs/day) Original Loss ________________________________________________________________________ 1 75.46 100.0 2 73.43 96.0 3 52.79 68.9 4 43.60 56.8 5 37.99 49.5 6 34.13 45.2 7 31.24 41.4 8 28.99 38.4 9 27.15 36.0 10 25.64 34.0 ______________________________________________________________________
Using Table 6 and the previous example where a conveyance loss of 32.6 cfs was determined for one day, the loss for the second day would be 96.0% of 32.6 cfs or 31.3 cfs given a constant flowrate. The conveyance loss for the third day would be 68% of 32.6 cfs or 22.5 cfs, given that the flowrate remained constant at 500 cfs.
Using Figure 12, the preceding process can be used to determine incremental conveyance loss for any increase in flowrate from 0 to 1950 cfs, but is most accurate between 300 and 600 cfs. A flow of 1950 cfs was chosen because it was approximately 50 percent higher than any flowrate measured during the study period. The practical value of Figure 12 is that hydrographers on the Greybull River can use it to quickly estimate a conveyance loss for any given increase in flowrate so that downstream users can be properly assessed that loss.
Figure 13. Greybull River Modeling Results Using Total Conveyance Loss to Determine Incremental Conveyance Loss.
WIND RIVER STUDY AREA
The period of analysis to be discussed in this thesis for the Wind River study area begins June 1, 1992 and ends September 30, 1992. Average daily diversion values were chosen for the same reasons indicated in discussion of the Greybull River study reach.
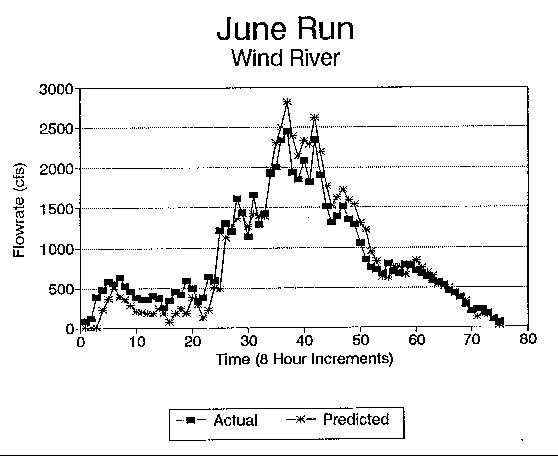
The first period in the study of the Wind River reach extended from June 15, 1992 to July 9, 1992. During this period of record, high flowrates were recorded and the model was calibrated to the actual flow records. Figure 15 illustrates the results of this calibration which shows that for high flowrates, the model simulated actual data moderately well. However, when the flow data immediately following the first study period were analyzed, a disparity was encountered. The modelled data indicated that the river was flowing on average approximately 100 cfs more at the downstream end of the study reach than could be accounted for by the model.
Figure 14. Greybull River Results, Total Conveyance Loss as a Percent of Original Conveyance Loss with Time.
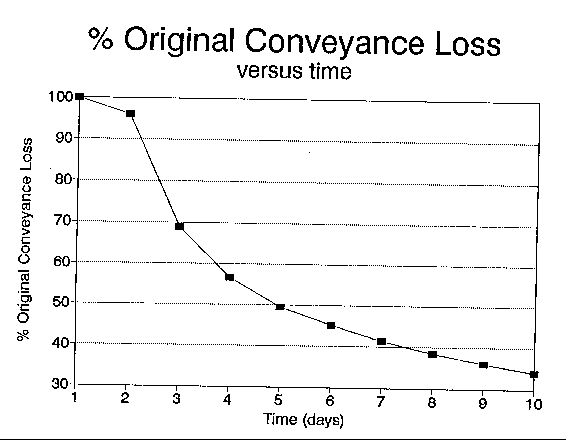
The problem was most likely with the actual data. The first problem uncovered with the actual data was that the Johnstowh ditch which diverts flow above the upper gaging station on the study reach actually returns ungaged flows directly into the Wind River in the study reach. The ditch diverts water from the Wind River approximately 200 yards upstream of the Kinnear gaging station and the wastewater re-enters the Wind River within the study reach possessing at times a significant amount of the flowrate that was originally diverted. Secondly a canal that starts in the Little Wind River drainage and ends up irrigating land within the Wind River drainage has a spill system that allows excess canal water to flow into the Wind River. This spill system was unknown when the study was initiated and was not brought to our attention until August, 1993. Water of an unknown flowrate was entering the Wind River within the study reach. At times this wastewater was estimated to be in the neighborhood of 60 to 80 cfs. The consequences of these ungaged flows is that flow measured at the Riverton gaging station was higher than a mass balance of the flows in the reach would indicate. This discovery caused the Wind River analysis for 1992 to be highly suspect and no further analyses were performed.
Figure 15. Wind River (June 1992) Model Calibration Comparison of Actual Flows and Modeled Flows.
It is important to note that while the J349 model successfully modelled the first study period for the Wind River reach, these results cannot be accepted as valid. The reason is that the high flowrates that were encountered during this study period masked the gains incurred in the study reach. Flows during this time period ranged between 2000 and 3500 cfs at the Riverton gage. A gain of 100 cfs during this time period is a small difference of between three and five percent of the total flowrate. However, the flowrate for much of the remainder of the summer averaged between 200 cfs and 500 cfs at the Riverton gage. A gain of 100 cfs during this time period results in a difference of between twenty and fifty percent. A difference in flowrate of five percent would be within the measurement accuracy for the gaging station. An error of twenty percent, however, is too extreme to say that model results are simulating flows in the Wind River. The study on the Wind River reach to estimate conveyance losses was suspended as a result of these discoveries. It will require another season of measurements which will include canal wastewater return flow measurements into the Wind River before estimates can be made on conveyance losses using the modelling approach presented. The model did, however, indicate the return flow problem.
94-05 Table of Contents
Water Resources Publications List
Water Resources Data System Library |
Water Resources Data System Homepage